Susan Norma, Candland Bromley and Dr. Larry L. Howell, Mechanical Engineering

The objective of this research was to investigate the stress and strain behavior of microscopic cantilevered polycrystallin silicon (polysilicon) beams. A knowledge of this behavior is necessary to accomplish reliable mechanical design of micro-electro-mechanical systems (MEMS).

In order to better understand the mechanical behavior of polysilicon at the microscopic level, a study was conducted to determine how the nominal strain at failure of a microscopic cantilevered beam is affected by variations in device geometry. Previous research has shown that significant scatter exists in data measuring the Young’s modulus and other material properties of polysilicon devices with microscopic dimensions (Mullen et al., 1997, Sharpe et al. 1997). In order to explain this scatter, a full factorial experiment was conducted to find whether device geometry affects the nominal strain at failure. The nominal strain was studied in order to eliminate the variation in Young’s modulus from the experiment.

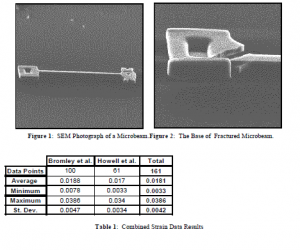
Microscopic cantilevered beams were designed combining all the possible variation of three lengths, three heights, two widths, and two stress concentrations. This yielded thirty-six unique beam designs. A scanning electron microscope (SEM) photograph of a representative microbeam is shown in Figure 1. These thirty-six beam designs were then randomized into three separate runs in order to eliminate any variation introduced by factors outside the control set. Of the 108 beams, eight were broken before they could be tested. Under an optical microscope, the researcher manually deflected the remaining 100 beams to failure with a microprobe. Figure 2 shows the fractured base of a microbeam. The deflection and failure process of each beam was recorded on video. The images were analyzed and the deflections were obtained using motion analysis software. Finite element analysis was used to convert the deflections to nominal stresses. Because the majority of the beam deflections exceeded small angle deflection, the FEA program employed nonlinear analysis in obtaining the nominal stress solution. Finally, the strain was calculated.

By examining the nominal strain data, the researchers found that the influence of geometry on nominal strain is insignificant. The strain data from this experiment were combined with the results of a previous study done at BYU. The strain results are summarized and the weighted totals are given in Table 1. Using a the formula

eo £ (eo)failure – 3*(standard deviation)

the researchers calculated a recommended maximum nominal strain of 0.0055; this strain can be used as a constraint in the design of MEMS devices.

References

• Mullen, R.L., Ballarini, R., Yin, Y., and Heuer, A. H., 1997, “Monte Carlo Simulation of Effective Elastic Constants of Polycrystalline Thin Films,” Acta mater., Vol. 45, No. 6, pp 2247- 2255.
• Sharpe, W.N., Yuan, B., and Vaidyanathan, R., 1997, “Measurements of Young’s Modulus, Poisson’s Ratio, and Tensile Strength of Polysilicon,” IEEE Micro Electro Mechanical Systems, pp. 424-429.