Taylor Killian and Dr. Tadd Truscott, Department of Mechanical Engineering
Goal
The purpose of the proposed research was to mathematically define and model the flow of fluid within a partially filled sphere. The goal of this modeling was to aid the study of the effects an enclosed fluid had on its boundary. We desired to demonstrate the movements of the fluid numerically. Professor Truscott’s laboratory observed that the rebound of a sphere, partially filled with fluid, is greatly reduced by the motion of the fluid inside the sphere and wanted to understand the dynamics of this event.
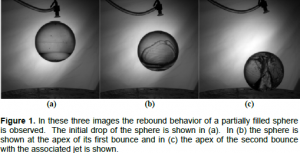
In Figure 1, the rebounding motion of a partially filled sphere can be observed. The impact of the first bounce results in a parabolic cavity-like formation of the fluid inside the sphere. This change in shape of the fluid surface coupled with the fluid interaction of the spherical boundary results a large jet forming after the second bounce. The rebound of the sphere is drastically reduced after the second bounce due to this fluid interaction. The initial hypothesis was that the formation of the fluid in the second image before the second bounce and formation of the powerful jet stabilizes the movement of the sphere. This hypothesis was validated through modeling and was verified through extensive study and research of the literature.
Approach
Initially we hoped to demonstrate the entire event of jet formation and, through that work, be able to infer the amount of fluid was moved and its velocity. This information would provide enough information to understand the amount of energy that was removed from the rebounding sphere by the fluid. As is common practice when modeling fluid interacting with a solid boundary, we tried to implement a method known as potential flow. This mathematical construct defines equations that direct the flow of an idealized fluid volume that is initially assigned a velocity and direction. Typically this type of modeling is used to demonstrate how fluid moves around obstructions, with the core of the mathematics used to define the placement and geometry of that obstruction. We hoped to adapt these common practices to our problem by defining the parabolic cavity-like formation as our boundary and show its collapse and resulting jet formation. The final step of our research proposal was to dynamically continue this model to show the fully formed jet and changed boundary shape, hoping to include the fluid motion in an enclosed area to reflect the actual system described above.
Results
After developing an initial model in MATLAB, a mathematical computer program, we were met with several obstacles of using potential flow modeling to demonstrate our experiments. We were able to show the beginnings of jet formation, shown in Figure 2, but were not
able to continue the simulation to show the cavity collapse and jet formation using the mathematics of potential flow. You may also note that the flow lines in Figure 2 do not accurately demonstrate how the fluid would flow with the presence of a spherical boundary representing the sphere.The primary obstacle, and one that we were not able to overcome, was that the mathematics of potential
flow modeling were derived to lend themselves to physical problems where the amount of fluid is unlimited and is not confined. The ideal potential flow modeling problem is that of a stream of fluid passing over and around a fixed object. The complexities of having a moving boundary with a confined amount of fluid proved to be too difficult to address.
Despite the disappointment of realizing that we were not able to model the fluid flow within a partially filled sphere, we pushed forward trying to form some analytical understanding of what was happening to the sphere that caused its rebound to be reduced by the inclusion of fluid. As we studied the collected data we decided to approach the problem from a perspective of the momentum transfer from the sphere to the fluid. This turned out to be an instructive that ultimately provided a great description of the overall behavior of the partially filled sphere. I was tasked to develop a model that would describe the exchange of energy from the falling sphere to the fluid. We succeeded in demonstrating that the great majority of the sphere’s energy it uses to rebound is lost to the fluid at the second bounce. While we were not able to definitively determine the specific dynamics of the fluid flow mathematically, it is now clear to us what role the fluid plays to reduce the rebound of the sphere.
Academic Outcome
We are currently drafting the results of our research to be published in the peer-reviewed journal Experiments in Fluids. As a result of the progress that we have made we have presented this research at academic conferences. We first created a poster describing our experiments and presented it at the Annual meeting of the American Physical Society-Division of Fluid Dynamics (APS-DFD) in San Diego,
November 2010. I presented our initial results at the BYU College of Physical and Mathematical Sciences Student Research Conference at the APS March Meeting in early 2011. A more complete and fuller presentation of our results was given at the 2011 APS-DFD meeting in Baltimore, MD.
Personal Outcome
The opportunity to struggle and succeed while working under the mentoring of Professor Truscott has been influential in my intellectual development. I intend to pursue a doctorate degree in Applied Mathematics with research involved in fluid dynamics as a result of the work that I was able to perform with Professor Truscott over these past 18 months and will continue to perform with him as I conclude my BYU education. I am grateful for the added funding that BYU’s Office of Research and Creative Activities provided to enable full attention on this work auxiliary to my BYU education.
References
- A. Antkowiak, N. Bremond, S. Le Dizes and E. Villermaux, “Short-term dynamics of a density interface following an impact,” J. Fluid Mech., vol. 577, pp. 241-250, 2007.
- R. Bergmann, D. van der Meer, M. Stijnman, M. Sandtke, A. Prosperetti and D. Lohse, “Giant Bubble Pinch-Off,” PRL, vol. 96, 2006.
- V. Duclaux, F. Caille, C. Duex, C. Ybert, L. Bocquet and C. Clanet, “Dynamics of transient cavities,” J. Fluid Mech., vol. 591, pp. 1-19, 2007.
- S. Gekle and J. M. Gordillo, “Generation and Breakup of Worthington Jets After Cavity Collapse.” J. Fluid Mech., 2010.
- S. Gekle, J. M. Gordillo, D. van der Meer and D. Lohse, “High-Speed Jet Formation after Solid Object Impact,” PRL, vol. 102, 2009.
- R. A. Ibrahim and V. N. Pilipchuk, “Recent Advances in liquid sloshing dynamics,” Appl Mech Rev, vol. 54, pp. 133-199, 2001.
- T. Truscott, B. Epps and A. Techet, “Unsteady forces on sphere during free-surface water entry,” J. Fluid Mech., 2010.
